Live Classroom Vignette
Written by: Dr. Christine von Renesse and Dr. Volker Ecke.
This blog contains a vignette from a “live classroom” or “immersive activity” for faculty during a workshop. We want to give the reader a sense of what it can look like to have faculty be students in a mini classroom experience. Toward the end we explain how we are running the reflection session after the live classroom experience, which ties the student experience to the teacher tools and the 4 pillars of inquiry-based learning.
The main goal of a live classroom is to describe and analyze the components of a particular inquiry classroom. To this end, a math facilitator teaches an inquiry class in which the participants are the students. The activity is created in a way that the participants can be their true mathematician selves and do not need to role play being students. After the math activity, a pedagogy facilitator helps the participants to reflect on the student experiences and analyze what the math facilitator did or did not do to run the class. With the live classroom, the participants co-create one particular representation of learning with inquiry that can be helpful for further discussions.
The scenario is written such that the modality could be in person or remote synchronous. We have run the activity in both modalities many times and the main differences show up in the choice of tools. While in person we would have sat around tables, we meet online in breakout groups. Instead of writing on paper or on boards, we use google docs and jamboards. For the random in-person connections, there are online ice-breakers and the chat function.
Vignette:
Prof Christine von Renesse is running the live classroom in this workshop. To set the stage, she explains that her model of inquiry is not supposed to be THE model or THE BEST teaching, but just one way of running a class with inquiry. “This will give us a shared experience of a class taught with inquiry so that we can talk about all the different aspects later on. You do not need to pretend to be students, you can be your true mathematician self. If you have brain space available you can think about what I am doing during class, but your priority right now is being a student.”
Norm Setting:
To think about how we work together, Prof von Renesse asks participants to share what they like when they collaborate with others and what they do not like. What they share can be synthesized in an informal list of norms for the day: either in writing or just spoken.
Group Work:
The workshop participants sit in groups around tables and work on the task: determine all possible values generated by the equation 3a+5b, when a,b≥0. They work for about 20 minutes while Prof. von Renesse rotates around, listening to the groups’ thinking, and maybe asking some probing questions or making a suggestion about how to record the findings. Just like in a classroom with students, some participants struggle, and some remember the problem and quickly find a conjecture and a proof. To the latter group, the facilitator gives an extension question: “What else are you curious about when you see this problem? What would you like to consider next”?
The group decides to change the numbers 3 and 5 to other numbers and observe the patterns. For the groups that struggle, Prof. von Renesse tries to decide if they are in a happy place of struggle or if they are getting too frustrated. One group has made a mistake that makes their conjecture incorrect and since they are already feeling frustrated, she invites them to compare notes with another group to check the list of numbers that can not be generated. This helps the struggling group back into more productive discussions. The facilitator is taking a few notes on a piece of paper to remember which of the 6 groups used which methods and in which order it may make sense to have them share their results.
Whole Class Discussion:
When Prof. von Renesse calls the whole group together, the first prompt makes everyone think: “What did you struggle with in this activity?” After a long pause, some volunteers said that they didn’t know how to get started. Some admitted that they could not remember a proof technique they were looking for, and some shared that they were nervous because they didn’t know what exactly was expected of them. Some had misread the prompt and kept a=b or didn’t allow a and b to be zero which caused some setbacks at first. Now Prof von Renesse asks one of the groups to share their thinking. The group presents their process work: they tried generating numbers at first, making lists of numbers you can get and some you can not get. Then they changed their thinking and made the conjecture that you can get all numbers eventually except for some smaller numbers. To prove their conjecture, they used a random big number and showed how you can find out how many 3s and how many 5’s can fit into the number: They first subtracted a multiple of 3 so that they get a number that ends in zero. Then they divided by 5. The facilitator asks if one of the other groups could explain what they had just learned from group 1, or if they could ask a question. A volunteer explains the process of division, but also has a question: “How do you know that this will work for any number?” This results in an interesting discussion about how to make the argument more rigorous. When 2 more groups share their proofs, participants are surprised by the differences in process, representations, and proofs.
The group that had chosen an extension question found a new conjecture about the last number that can not be generated for any combination xa+yb - but they were not able to find a proof yet. The room is buzzing with positive energy and no one is ready to stop when Prof von Renesse announces that we are done doing math now. “I know this is hard to let go of. The rest has to wait for lunch break.” It is not easy to convince mathematicians to let go of a math problem and it takes a few minutes before the group is willing to move on.
Reflection:
To start the reflection phase, Prof von Renesse mentions that it is sometimes not easy to work with peers. She invites participants to be as vulnerable as they dare when they are reflecting on their student experience. Immediately someone speaks up: “It felt weird not to know the answer and feeling lost, I was wondering what exactly was expected of me.” Another adds on: “I felt nervous that everyone else in the group may get the answer much faster than me and that I will feel stupid or left behind. This is probably how my students feel all the time!” The facilitator appreciates the vulnerability and acknowledges that it is not easy to do math together, especially when you do not know the other people yet. Other participants share that they had a lot of fun with the task and enjoyed getting lost in the reasoning. Many participants look thoughtful as they are considering what their students may feel when they are challenging them with new tasks.
After a few minutes, Prof von Renesse invites the participants to take their “student hats” off and put their “teacher hats” back on. The next prompt invites them to reflect on the experience again, but this time as teachers: “What did you observe Prof von Renesse do? What didn’t you observe her do? Why did she make these choices?” As participants mention many of the aspects of a class run with inquiry, the aspects are being recorded for everyone to see and access later on. Some deeper probing is necessary: “Why did I ask you what was hard about the activity in the beginning of the whole class discussion?” After a long pause someone offers “because people may feel better when they can say what they struggled with?” “Yes, but what about the people that just listened, what does it do for them?” “Oh, if they struggled as well they now feel better about it!.” “Absolutely. It normalizes feeling stuck, making mistakes, being confused - and these are essential for learning with inquiry.”
Someone mentions that Prof von Renesse asked specific groups to share their thinking in a particular order and used “talk moves” to connect them. “How do you decide in which order to go? Does every group have to share?” Instead of answering the question, the facilitator gives the question back to the group: “What do you think? How could I have made that choice?” and records the answers.
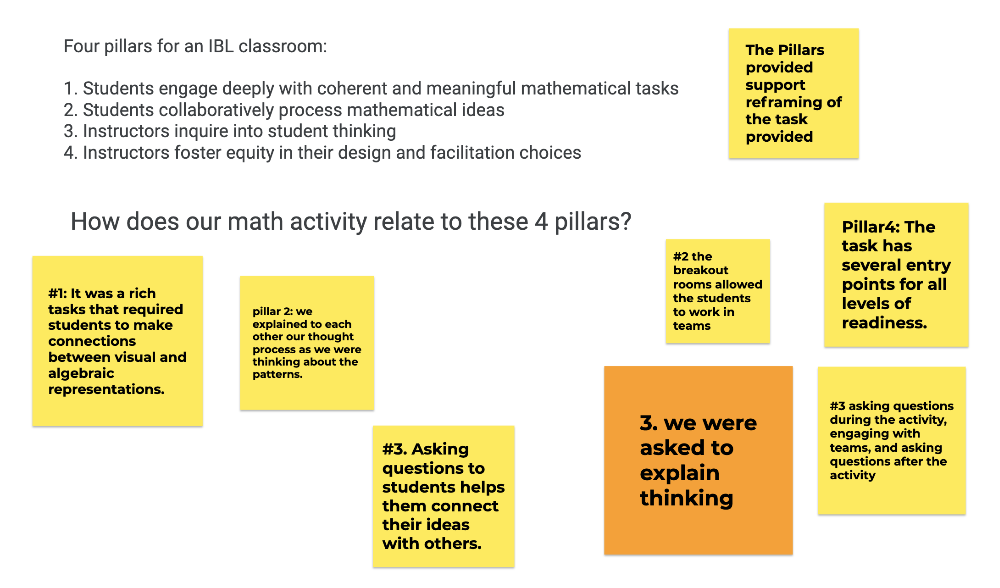
Toward the end of the reflection phase, Prof. von Renesse displays the 4 pillars of inquiry-based learning, see image below. Participants are tasked to provide examples for each of the pillars from the live classroom activity. This provides the opportunity to think more deeply about “good tasks” as well as equity in the classroom.
The last 10 minutes are open for any questions from the participants. They range from assessment and grading strategies, over finding curriculum, to collaborating with colleagues.
Resources:
See also this blog about transformative change in teaching and our workshop video page.